see rotations theory
The angular momentum is the linear momentum times its distance from the axis
of rotation.
When working out angular momentum its no use just summing the angular momentum's
around the individual centres-of-mass of each body
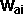 *
*  +
+ 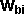 *
* 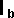 =
= 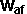 *
*  +
+ 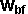 *
* 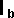
where:
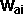 = initial angular speed of
body A = initial angular speed of
body A |
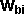 = initial angular speed of
body B = initial angular speed of
body B |
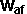 = final angular speed of body
A = final angular speed of body
A |
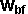 = final angular speed of body
B = final angular speed of body
B |
 = second moment of mass about
c-of-m of A = second moment of mass about
c-of-m of A  |
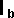 = second moment of mass about
c-of-m of B = second moment of mass about
c-of-m of B  |
Example 1
Imagine two bodies A and B of the same shape and mass. A is initially at rest,
B is traveling toward A with no initial spin. So since they both started with
with zero spin we might expect them to end up with equal and opposite spin.
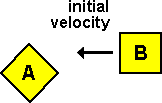
However that is not the case because A is hit in line with its own c-of-m so
it gets no rotational component, however object B is not hit in line with its
c-of-m so it will get a spin component.

Therefore it is the angular momentum about the c-of-m OF THE WHOLE SYSTEM that
is conserved?
I think this means that if, the point of impact is different from whole system
c-of-m, then we need an extra term to include this extra rotational component
about the system c-of-m.
But does this work? Consider another example:
Example 2
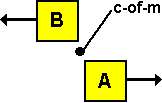
Two bodies with the same shape and mass, passing each other with equal and
opposite speed and offset by distance r. Therefore the centre of mass of the
whole system is stationary in the middle. Since the bodies are moving away,
the moment of mass is increasing and the angle of rotation about the c-of-m
is changing. But is the overall angular momentum remaining constant?
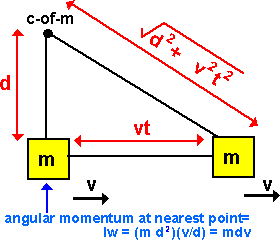
From the above diagram the angular momentum at the nearest point to the c-of-m
= m d v
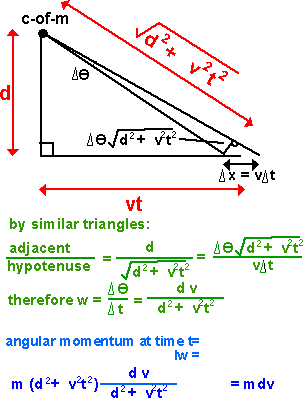
From the above after time t the angular momentum is still m d v
So angular momentum is conserved.
Thanks to Leo Wibberly for sending me this solution.
|
metadata block |
|
|
| see also: |
go to forces page
|
| Correspondence about this page |
|
|
Book Shop - Further reading.
Where I can, I have put links to Amazon for books that are relevant to
the subject, click on the appropriate country flag to get more details
of the book or to buy it from them. |






 Physics for Game Developers - Assumes a knowledge of vectors, Matrix and trigonometry
(the book has a one page introduction to quatnions). The book introduces Newtons
laws but it does assume a basic knowledge physics. It covers Kinematics, Force,
Kinetics, Collision (detection), Projectiles, Aircraft, Ships, Hovercraft, Cars,
Real-time, 2D rigid body, Collision Response, Rigid body rotation, 3D rigid body,
multiple bodies in 3D and particles. (I cant find a general formula for collision
response which combines linear and rotation, but there may be something in the
code included?). If you don't have the prerequisite knowledge of Matrices etc.
you may want to get the Mathematics for 3D
Game programming book first.
Physics for Game Developers - Assumes a knowledge of vectors, Matrix and trigonometry
(the book has a one page introduction to quatnions). The book introduces Newtons
laws but it does assume a basic knowledge physics. It covers Kinematics, Force,
Kinetics, Collision (detection), Projectiles, Aircraft, Ships, Hovercraft, Cars,
Real-time, 2D rigid body, Collision Response, Rigid body rotation, 3D rigid body,
multiple bodies in 3D and particles. (I cant find a general formula for collision
response which combines linear and rotation, but there may be something in the
code included?). If you don't have the prerequisite knowledge of Matrices etc.
you may want to get the Mathematics for 3D
Game programming book first.






 Classical Dynamics of Particles and Systems - I have not reviewed this book, so
I would be interested to hear if it is useful? I would also be interested to hear
if there are any other books that may be of interest to readers of this page.
Classical Dynamics of Particles and Systems - I have not reviewed this book, so
I would be interested to hear if it is useful? I would also be interested to hear
if there are any other books that may be of interest to readers of this page.






 Engineering Mechanics - Includes Statics book and dynamics book below..
Engineering Mechanics - Includes Statics book and dynamics book below..






 Engineering Mechanics Vol 2: Dynamics - Gives theory for rigid dynamics, aims
to allow prediction of effects of force and motion. Includes rotating frame
of reference. Lots of colour diagrams, I guess its college / University level.
Engineering Mechanics Vol 2: Dynamics - Gives theory for rigid dynamics, aims
to allow prediction of effects of force and motion. Includes rotating frame
of reference. Lots of colour diagrams, I guess its college / University level.
|
|
Commercial Software Shop
Where I can, I have put links to Amazon for commercial software, not
directly related to the software project, but related to the subject being
discussed, click on the appropriate country flag to get more details of
the software or to buy it from them. |

 The 3D Gamemaker
The 3D Gamemaker
|
This site may have errors. Don't use for critical systems.
![]() *
* ![]() +
+ ![]() *
* ![]() =
= ![]() *
* ![]() +
+ ![]() *
* ![]()










